How To Find Empirical Formula Of Vitamin C

How To Find Empirical Formula Of Vitamin C

3.5: Empirical Formulas from Analysis

1. Last updated

2. Save as PDF

Page ID

21710

Learning Objectives

• To understand the definition and difference between empirical formulas and chemical formulas
• To understand how combustion analysis can be used to identify chemical formulas

Chemical formulas tell you how many atoms of each element are in a compound, and empirical formulas tell you the simplest or most reduced ratio of elements in a compound. If a compound's chemical formula cannot be reduced any more, then the empirical formula is the same as the chemical formula. Combustion analysis can determine the empirical formula of a compound, but cannot determine the chemical formula (other techniques can though). Once known, the chemical formula can be calculated from the empirical formula.

Empirical Formulas

An empirical formula tells us the relative ratios of different atoms in a compound. The ratios hold true on the molar level as well. Thus, H₂O is composed of two atoms of hydrogen and 1 atom of oxygen. Likewise, 1.0 mole of H₂O is composed of 2.0 moles of hydrogen and 1.0 mole of oxygen. We can also work backwards from molar ratios since if we know the molar amounts of each element in a compound we can determine the empirical formula.

Example \(\PageIndex{1}\): Mercury Chloride

Mercury forms a compound with chlorine that is 73.9% mercury and 26.1% chlorine by mass. What is the empirical formula?

Solution

Let's say we had a 100 gram sample of this compound. The sample would therefore contain 73.9 grams of mercury and 26.1 grams of chlorine. How many moles of each atom do the individual masses represent?

For Mercury:

\[(73.9 \;g) \times \left(\dfrac{1\; mol}{200.59\; g}\right) = 0.368 \;moles \nonumber\]

For Chlorine:

\[(26.1\; g) \times \left(\dfrac{1\; mol}{35.45\; g}\right) = 0.736\; mol \nonumber\]

What is the molar ratio between the two elements?

\[\dfrac{0.736 \;mol \;Cl}{0.368\; mol\; Hg} = 2.0 \nonumber\]

Thus, we have twice as many moles (i.e. atoms) of \(\ce{Cl}\) as \(\ce{Hg}\). The empirical formula would thus be (remember to list cation first, anion last):

\[\ce{HgCl2} \nonumber\]

Chemical Formula from Empirical Formula

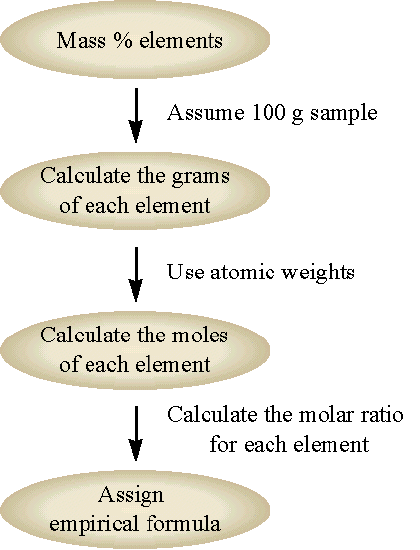
The chemical formula for a compound obtained by composition analysis is always the empirical formula. We can obtain the chemical formula from the empirical formula if we know the molecular weight of the compound. The chemical formula will always be some integer multiple of the empirical formula (i.e. integer multiples of the subscripts of the empirical formula). The general flow for this approach is shown in Figure \(\PageIndex{1}\) and demonstrated in Example \(\PageIndex{2}\).

Figure \(\PageIndex{1}\): The general flow chart for solving empirical formulas from known mass percentages.

Example \(\PageIndex{2}\): Ascorbic Acid

Vitamin C (ascorbic acid) contains 40.92 % C, 4.58 % H, and 54.50 % O, by mass. The experimentally determined molecular mass is 176 amu. What is the empirical and chemical formula for ascorbic acid?

Solution

Consider an arbitrary amount of 100 grams of ascorbic acid, so we would have:

• 40.92 grams C
• 4.58 grams H
• 54.50 grams O

This would give us how many moles of each element?

• Carbon

\[ (40.92\; \cancel{g\; C}) \times \left( \dfrac{1\; mol\; C}{12.011\; \cancel{g\; C}} \right) = 3.407\; mol \; C \nonumber \]

• Hydrogen

\[ (4.58\; \cancel{g\; H}) \times \left( \dfrac{1\; mol\; H}{1.008\; \cancel{g\; H}} \right) = 4.544\; mol \;H \nonumber \]

• Oxygen

\[ (54.50\; \cancel{g\; O}) \times \left( \dfrac{1\; mol\; O}{15.999\; \cancel{g\; O}} \right) = 3.406\; mol \; O \nonumber \]

Determine the simplest whole number ratio by dividing by the smallest molar amount (3.406 moles in this case - see oxygen):

• Carbon

\[ C= \dfrac{3.407\; mol}{3.406\; mol} \approx 1.0 \nonumber\]

• Hydrogen

\[ C= \dfrac{4.5.44\; mol}{3.406\; mol} = 1.0 \nonumber\]

• Oxygen

\[ C= \dfrac{3.406\; mol}{3.406\; mol} = 1.0 \nonumber\]

The relative molar amounts of carbon and oxygen appear to be equal, but the relative molar amount of hydrogen is higher. Since we cannot have "fractional" atoms in a compound, we need to normalize the relative amount of hydrogen to be equal to an integer. 1.333 would appear to be 1 and 1/3, so if we multiply the relative amounts of each atom by '3', we should be able to get integer values for each atom.

C = (1.0)*3 = 3

H = (1.333)*3 = 4

O = (1.0)*3 = 3

or

\[\ce{C3H4O3}\]

This is our empirical formula for ascorbic acid.

What about the chemical formula? We are told that the experimentally determined molecular mass is 176 amu . What is the molecular mass of our empirical formula?

(3*12.011) + (4*1.008) + (3*15.999) = 88.062 amu

The molecular mass from our empirical formula is significantly lower than the experimentally determined value. What is the ratio between the two values?

(176 amu/88.062 amu) = 2.0

Thus, it would appear that our empirical formula is essentially one half the mass of the actual molecular mass. If we multiplied our empirical formula by '2', then the molecular mass would be correct. Thus, the actual chemical formula is:

2* C₃H₄O₃ = C₆H₈O₆

Empirical Formulas: https://youtu.be/UqHI3gmgQOA

Combustion Analysis

When a compound containing carbon and hydrogen is subject to combustion with oxygen in a special combustion apparatus all the carbon is converted to CO₂ and the hydrogen to H₂O (Figure \(\PageIndex{2}\)). The amount of carbon produced can be determined by measuring the amount of CO₂ produced. This is trapped by the sodium hydroxide, and thus we can monitor the mass of CO₂ produced by determining the increase in mass of the CO₂ trap. Likewise, we can determine the amount of H produced by the amount of H₂O trapped by the magnesium perchlorate.

Figure \(\PageIndex{2}\): Combustion analysis apparatus

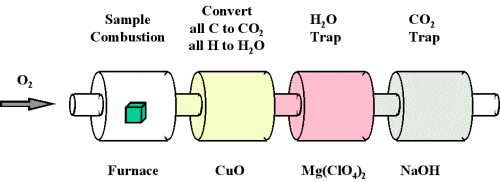
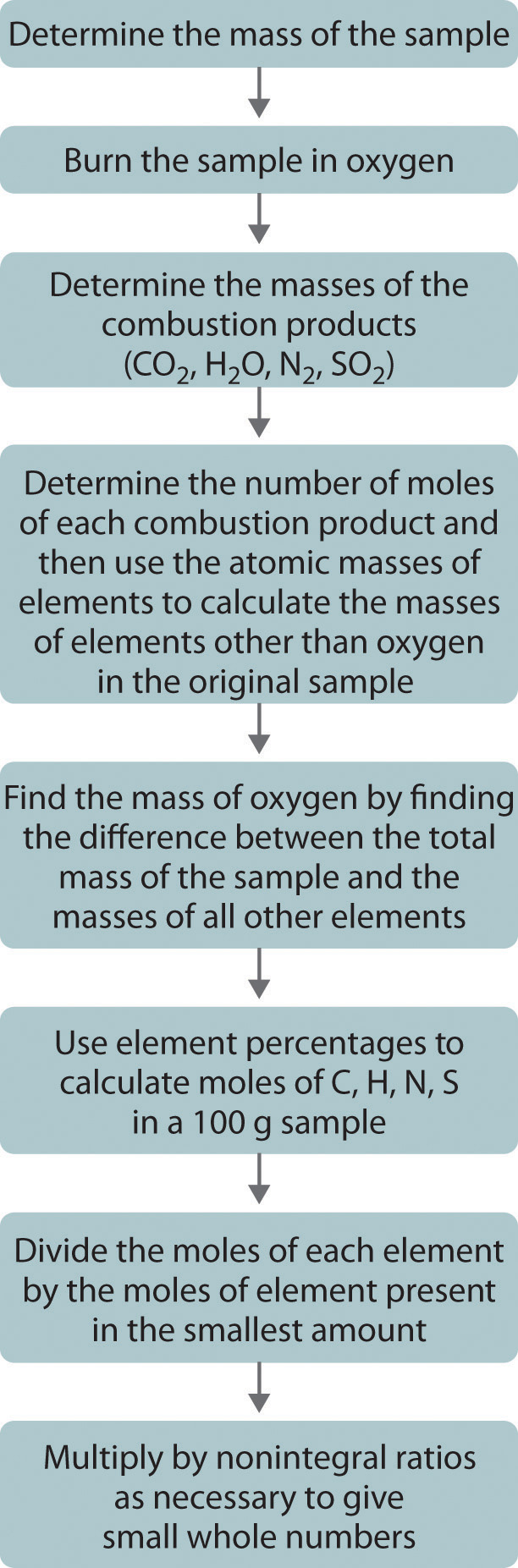
One of the most common ways to determine the elemental composition of an unknown hydrocarbon is an analytical procedure called combustion analysis. A small, carefully weighed sample of an unknown compound that may contain carbon, hydrogen, nitrogen, and/or sulfur is burned in an oxygen atmosphere,Other elements, such as metals, can be determined by other methods. and the quantities of the resulting gaseous products (CO₂, H₂O, N₂, and SO₂, respectively) are determined by one of several possible methods. One procedure used in combustion analysis is outlined schematically in Figure \(\PageIndex{3}\) and a typical combustion analysis is illustrated in Examples \(\PageIndex{3}\) and \(\PageIndex{4}\).

Figure \(\PageIndex{3}\): Steps for Obtaining an Empirical Formula from Combustion Analysis. (CC BY-NC-SA; anonymous)

Example \(\PageIndex{3}\): Combustion of Isopropyl Alcohol

What is the empirical formulate for isopropyl alcohol (which contains only C, H and O) if the combustion of a 0.255 grams isopropyl alcohol sample produces 0.561 grams of CO₂ and 0.306 grams of H₂O?

Solution

From this information quantitate the amount of C and H in the sample.

\[ (0.561\; \cancel{g\; CO_2}) \left( \dfrac{1 \;mol\; CO_2}{44.0\; \cancel{g\;CO_2}}\right)=0.0128\; mol \; CO_2 \]

Since one mole of CO₂ is made up of one mole of C and two moles of O, if we have 0.0128 moles of CO₂ in our sample, then we know we have 0.0128 moles of C in the sample. How many grams of C is this?

\[ (0.0128 \; \cancel{mol\; C}) \left( \dfrac{12.011\; g \; C}{1\; \cancel{mol\;C}}\right)=0.154\; g \; C \]

How about the hydrogen?

\[ (0.306 \; \cancel{g\; H_2O}) \left( \dfrac{1\; mol \; H_2O}{18.0\; \cancel{g \;H_2O}}\right)=0.017\; mol \; H_2O \]

Since one mole of H₂O is made up of one mole of oxygen and two moles of hydrogen, if we have 0.017 moles of H₂O, then we have 2*(0.017) = 0.034 moles of hydrogen. Since hydrogen is about 1 gram/mole, we must have 0.034 grams of hydrogen in our original sample.

When we add our carbon and hydrogen together we get:

0.154 grams (C) + 0.034 grams (H) = 0.188 grams

But we know we combusted 0.255 grams of isopropyl alcohol. The 'missing' mass must be from the oxygen atoms in the isopropyl alcohol:

0.255 grams - 0.188 grams = 0.067 grams oxygen

This much oxygen is how many moles?

\[ (0.067 \; \cancel{g\; O}) \left( \dfrac{1\; mol \; O}{15.994\; \cancel{g \;O}}\right)=0.0042\; mol \; O \]

Overall therefore, we have:

• 0.0128 moles Carbon
• 0.0340 moles Hydrogen
• 0.0042 moles Oxygen

Divide by the smallest molar amount to normalize:

• C = 3.05 atoms
• H = 8.1 atoms
• O = 1 atom

Within experimental error, the most likely empirical formula for propanol would be \(C_3H_8O\)

Example \(\PageIndex{4}\): Combustion of Naphalene

Naphthalene, the active ingredient in one variety of mothballs, is an organic compound that contains carbon and hydrogen only. Complete combustion of a 20.10 mg sample of naphthalene in oxygen yielded 69.00 mg of CO₂ and 11.30 mg of H₂O. Determine the empirical formula of naphthalene.

Given: mass of sample and mass of combustion products

Asked for: empirical formula

Strategy:

1. Use the masses and molar masses of the combustion products, CO₂ and H₂O, to calculate the masses of carbon and hydrogen present in the original sample of naphthalene.
2. Use those masses and the molar masses of the elements to calculate the empirical formula of naphthalene.

Solution:

A Upon combustion, 1 mol of \(\ce{CO2}\) is produced for each mole of carbon atoms in the original sample. Similarly, 1 mol of H₂O is produced for every 2 mol of hydrogen atoms present in the sample. The masses of carbon and hydrogen in the original sample can be calculated from these ratios, the masses of CO₂ and H₂O, and their molar masses. Because the units of molar mass are grams per mole, we must first convert the masses from milligrams to grams:

\[ mass \, of \, C = 69.00 \, mg \, CO_2 \times {1 \, g \over 1000 \, mg } \times {1 \, mol \, CO_2 \over 44.010 \, g \, CO_2} \times {1 \, mol C \over 1 \, mol \, CO_2 } \times {12.011 \,g \over 1 \, mol \, C} \]

\[ = 1.883 \times 10^{-2} \, g \, C \]

\[ mass \, of \, H = 11.30 \, mg \, H_2O \times {1 \, g \over 1000 \, mg } \times {1 \, mol \, H_2O \over 18.015 \, g \, H_2O} \times {2 \, mol H \over 1 \, mol \, H_2O } \times {1.0079 \,g \over 1 \, mol \, H} \]

\[ = 1.264 \times 10^{-3} \, g \, H \]

B To obtain the relative numbers of atoms of both elements present, we need to calculate the number of moles of each and divide by the number of moles of the element present in the smallest amount:

\[ moles \, C = 1.883 \times 10^{-2} \,g \, C \times {1 \, mol \, C \over 12.011 \, g \, C} = 1.568 \times 10^{-3} \, mol C \]

\[ moles \, H = 1.264 \times 10^{-3} \,g \, H \times {1 \, mol \, H \over 1.0079 \, g \, H} = 1.254 \times 10^{-3} \, mol H \]

Dividing each number by the number of moles of the element present in the smaller amount gives

\[H: {1.254\times 10^{−3} \over 1.254 \times 10^{−3}} = 1.000 \, \, \, C: {1.568 \times 10^{−3} \over 1.254 \times 10^{−3}}= 1.250\]

Thus naphthalene contains a 1.25:1 ratio of moles of carbon to moles of hydrogen: C_1.25H_1.0. Because the ratios of the elements in the empirical formula must be expressed as small whole numbers, multiply both subscripts by 4, which gives C₅H₄ as the empirical formula of naphthalene. In fact, the chemical formula of naphthalene is C₁₀H₈, which is consistent with our results.

Exercise \(\PageIndex{4}\)

1. Xylene, an organic compound that is a major component of many gasoline blends, contains carbon and hydrogen only. Complete combustion of a 17.12 mg sample of xylene in oxygen yielded 56.77 mg of CO₂ and 14.53 mg of H₂O. Determine the empirical formula of xylene.
2. The empirical formula of benzene is CH (its chemical formula is C₆H₆). If 10.00 mg of benzene is subjected to combustion analysis, what mass of CO₂ and H₂O will be produced?

Answer a

The empirical formula is C₄H₅. (The chemical formula of xylene is actually C₈H₁₀.)

Answer b

33.81 mg of CO₂; 6.92 mg of H₂O

Combustion Analysis: https://youtu.be/A7ZjM9U5XAc

How To Find Empirical Formula Of Vitamin C

Source: https://chem.libretexts.org/Bookshelves/General_Chemistry/Map%3A_Chemistry_-_The_Central_Science_(Brown_et_al.)/03._Stoichiometry%3A_Calculations_with_Chemical_Formulas_and_Equations/3.5%3A_Empirical_Formulas_from_Analysis